Aerodynamics Analysis based on CFD can Provide Efficient Model Screening
Making changes to an airplane configuration can be expensive and sometimes dangerous. One method to reduce the expense is to test the proposed configurations in all possible modes of operations. Testing can be time consuming and has a natural enemy called "the deadline". Aerodynamics analysis methods based on computational fluid dynamics (CFD) methods can reduce testing time by rapidly screening models and pre-selecting only the promising ones for further testing (wind tunnels, scale models & flight testing).
Stallion 3D Automatic Gridding Dramatically Increases the Number of Models
That can be Tested Before the Deadline
If the goal of the design is to increase the load carrying capacity of the airplane, then each design iteration should be tested over a range of angles of attack to determine lift and the angle of attack for maximum lift.
Good Understanding of the Results can be Gained by Studying
Increasingly Complex Aerodynamic Models.
A good way to gain confidence (even in conceptual studies) is to study increasingly complex analytical and geometric models. The above graph starts with an airfoil analysis (using VisualFoil 5.0) and escalates using MultiSurface Aerodynamics (vortex lattice model for wing-tail) and Stallion 3D (Euler model for analyzing the full complex geometry).
MultiSurface Aerodynamics is an Interactive Software Based on the Vortex Lattice Method
for Quick 3-D Analysis of Complex Systems of Lifting Surfaces.
If your goal is to add equipment or payload capacity to the aircraft, then you must find the neutral point (or aerodynamic center) for each proposed model. The neutral point of the aircraft is defined as the position where Cm, the pitching moment coefficient, does not vary with a change in angle of attack (or dCm/d(AoA) = 0). To get a good understanding of this, one can plot Cm taken about a number of locations and plot each location as a function of angle of attack. To gain confidence in your analysis tool, you should use experience and a variety of methods to arrive at comparable results.
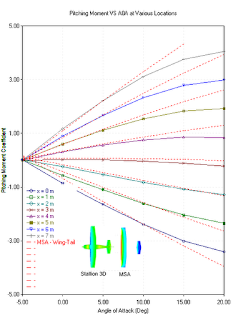
Cm vs AoA Taken About Various Positions from the Aircraft's Nose. The Stallion 3D Model
Compares Well with the MultiSurface Aerodynamics (MSA) Model.
The above graph shows that the neutral point is located about 3 meters from the nose of the aircraft. Here the slope of the Cm vs. Angle of Attack curve is zero. Ahead of the neutral point (0 < x < 3) m, the slope of the moment is negative. This means that if the center of gravity (CG) is located in this region, an increase in angle of attack (due to a gust for example) will be righted by an opposing moment about the CG. However, if the CG is located aft of the neutral point (3 < x < 8) m, then an increase in the angle of attack will be exacerbated by an enhancing moment action about the CG (this is not stable).
Another item to note is that as the CG is placed increasingly ahead of the neutral point, the restoring moment becomes larger. The distance between the CG and neutral point is known as the static margin. Increasing the static margin increases stability, however, the righting moment reduces the ability to control the aircraft. The design goal is to provide good stability with smaller control surfaces.
Studying the moment coefficients shows that in its current configuration, our aircraft will fly at an angle close to the zero-lift angle of attack. This is because the horizontal tail in the model (taken from NASA's Vehicle Sketch Pad program) is at zero angle of attack.
The Horizontal Tail Uses a Symmetric Airfoil at Zero Angle of Attack
To change the trim angle of the aircraft, we can control the angle of the horizontal tail surface. We assume that the CG is located 2 meters from the nose of the aircraft. The figure below shows that the aircraft will fly at angles of attack of -3 degrees, 0.7 degrees, 4.4 degrees for tail angle setting of 0.0, -5.0 and -10.0 degrees respectively.
MSA Analysis to Determine the Trim Angle of Attack of the Wing-Tail Model.
The fuselage shape and external components (stores and landing gears) can increase the drag of the airplane. The figure below compares the pressure drag computed by Stallion 3D against the profile and induced drag of the wing-tail configuration computed using MSA.
Drag Comparison of Two Models
The pressure drag of the fuselage, wing and struts adds to the total drag of the wing and tail. The goal of the design is to move the blue curve towards the green one. The green curve is the best that can be due given the wing planform shape and airfoil selection.
The results were complied after executing 6 cases in Stallion 3D with each run containing upwards of 700,000 cells. The the computations required about 8 to 10 hours per case on an HP laptop (2.4 gigahertz processor). The Stallion 3D floating licenses allow users to run the program on all available computers and processors. The time to set each case in Stallion 3D took less than one minute. The grid generation was automatic.
Stallion 3D was run at 6-different Angles of Attack for this Study
The cases ran in MultiSurface Aerodynamics were executed in less than one minute.
The above exercise involved longitudinal static considerations. We can repeat the exercise for lateral stability considerations. The above procedure can be carried out for design iterations and we could gain a good understanding of the behavior of the design before it advances to further testing.
Related Reading:
1. How to Enter and Analyze a Wing (in Stallion 3D): http://hanleyinnovations.com/winganalysis.pdf
2. NASA Vehicle SketchPad Page: http://vspmanual.webs.com/
3. Cessna 172 Performance Analysis by Temporal Images: http://www.temporal.com.au/aero.htm
4. Aerodynamics of a Circular Planform Airplane: http://www.eng.auburn.edu/organizations/AIAA/files/papers/2008/masters/Recktenwald_2008.pdf
Please email or call me at (352) 240-3658 if you have any questions.
Thanks for reading.